The unit circle | Maths | Quizalize & Oak National Academy

Starter quiz

In a right triangle, if the hypotenuse is 37.5 cm and a second side is 30 cm, what is the length of the third side? (Use a calculator to help you.)

22.5 cm ✓

34 cm

25 cm
Which of the following statements is true for these triangles?

The triangles are similar. ✓

The triangles are not similar.

It is not possible to know whether the triangles are similar or not.
An isosceles triangle has an internal angle of 50ᵒ, what might the other two angles be?

50ᵒ, 80ᵒ ✓

65ᵒ, 65ᵒ ✓

50ᵒ, 65ᵒ
In a right triangle, if the shortest sides are 36 cm and 48 cm, what is the length of the hypotenuse? (Use a calculator to help you.)

65 cm

60 cm ✓

62 cm
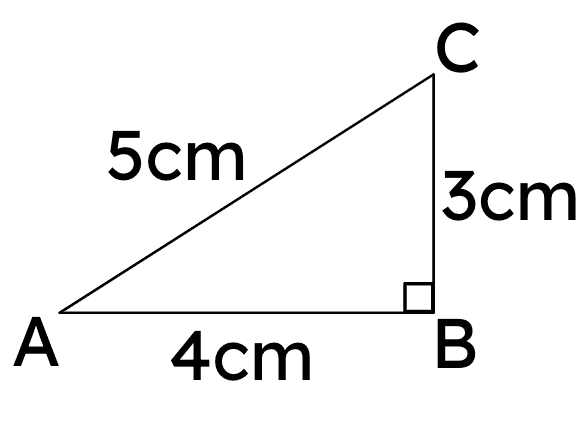Would a triangle ABC with sides AB = 10cm, BC = 7.5cm, AC = 12.5cm be similar to the one shown in the diagram?

Yes ✓

No
For this pair of triangles, can you determine whether they are similar without using side lengths?

Yes, because their three angles correspond. ✓

No because you only know two angles.

No, because you always need a side and two angles.

Exit quiz

What is the value of θ for sin(θ) = 1?

90ᵒ ✓

45ᵒ

50ᵒ

30ᵒ
What is the value of θ for tan(θ) = 1?

45ᵒ ✓

90ᵒ

30ᵒ

0ᵒ
What is the value of θ for cos(θ) = 0?

90ᵒ ✓

45ᵒ

30ᵒ

0ᵒ
What is the value of θ for sin(θ) = cos(θ)?

45ᵒ ✓

90ᵒ

30ᵒ

0ᵒ
What is the value of θ for sin(θ) = tan(θ)?

0ᵒ ✓

45ᵒ

90ᵒ
What is cos(60ᵒ)?

0.5 ✓

0.1

0

1

Worksheet

Loading worksheet ...

Presentation

Loading presentation ...

Video

Lesson Details

Key learning points

The unit circle is a circle with a radius of one.
The unit circle is centered on the origin.
The sine of an angle is the y-coordinate of the point where the radius has been rotated through that angle.
The cosine of an angle is the x-coordinate of the point where the radius has been rotated through that angle.
The tangent of an angle is the length of the side opposite the angle along the tangent at x = 1 to the unit circle.

Common misconception

When reading the values of the trigonometric functions during the explanation slides and the tasks, pupils may think that all the values taken from the graphs are fully accurate.

Explain that many of the values from the trigonometric functions have digits beyond the second decimal place. However, two decimal places is a reasonable degree of accuracy for reading values from the graphs during this lesson.

Keywords

Trigonometric functions - Trigonometric functions are commonly defined as ratios of two sides of a right-angled triangle for a given angle.
Sine function - The sine of an angle (sin(θ°)) is the y-coordinate of point P on the triangle formed inside the unit circle.
Cosine function - The cosine of an angle (cos(θ°)) is the x-coordinate of point P on the triangle formed inside the unit circle.
Tangent function - The tangent of an angle (tan(θ°)) is the y-coordinate of point Q on the triangle which extends from the unit circle.